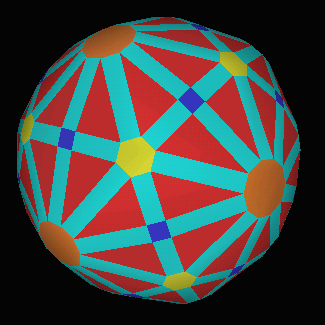
Illustrated here is a "transpolyhedron" which is based on the truncated icosidodecahedron and its dual. But the illustration can't capture the fact that when you view it, the different faces are continuously growing and shrinking
Transpolyhedron
is a term coined by Haresh Lalvani (see the references)
to describe a transitional form, intermediate between a polyhedron and
its dual. They display the duality relationship
in a very graphic manner, related to, but distinct from the way that a
compound of a polyhedron and its dual
displays the relationship.
A transpolyhedron contains all the faces of a given polyhedron, plus all the faces of its dual, plus a set of connecting rectangles---one for each edge of the original and/or dual. The relative sizes of the original and dual faces are adjustable, with the rectangles chosen to fill the intervening spaces. In the illustration, the decagons, hexagons, and squares derive from the original truncated icosidodecahedron; the triangles are from the dual; and the greenish-blue rectangles fill the gaps.
This page presents transpolyhedra in a way which is not just transitional, but also transformational. All these objects are defined with inherent movement, which causes them to oscillate between the original form and the dual form, displaying all the intermediate transpolyhedral forms.
Note: To start the transformation, click on the initial polyhedron within the viewer. You can spin it as it transforms, by dragging in the background sky area.
Platonic Solids:
- trans-tetrahedron --- transforms between a tetrahedron and its dual tetrahedron, momentarily passing through an intermediate cuboctahedron.
- trans-cube --- transforms between a cube and its dual octahedron, momentarily passing through an intermediate rhombicuboctahedron.
- trans-dodecahedron --- transforms between a dodecahedron and its dual icosahedron, momentarily passing through an intermediate rhombicosidodecahedron.
Archimedean Solids:
- trans truncated tetrahedron --- transforms between a truncated tetrahedron and its dual triakistetrahedron.
- trans cuboctahedron --- transforms between a cuboctahedron and its dual rhombic dodecahedron.
- trans truncated octahedron --- transforms between a truncated octahedron and its dual tetrakishexahedron.
- trans truncated cube --- transforms between a truncated cube and its dual triakisoctahedron.
- trans rhombicuboctahedron --- transforms between a rhombicuboctahedron and its dual trapezoidal icositetrahedron.
- trans truncated cuboctahedron --- transforms between a truncated cuboctahedron and its dual disdyakisdodecahedron.
- trans snub cube --- transforms between a snub cube and its dual pentagonal icositetrahedron.
- trans icosidodecahedron --- transforms between a icosidodecahedron and its dual rhombic triacontahedron.
- trans truncated icosahedron --- transforms between a truncated icosahedron and its dual pentakisdodecahedron.
- trans truncated dodecahedron --- transforms between a truncated dodecahedron and its dual triakisicosahedron.
- trans rhombicosidodecahedron --- transforms between a rhombicosidodecahedron and its dual trapezoidal hexecontahedron.
- trans truncated icosidodecahedron --- transforms between a truncated icosidodecahedron and its dual disdyakistriacontahedron.
- trans snub dodecahedron --- transforms between a snub dodecahedron and its dual pentagonal hexecontahedron.