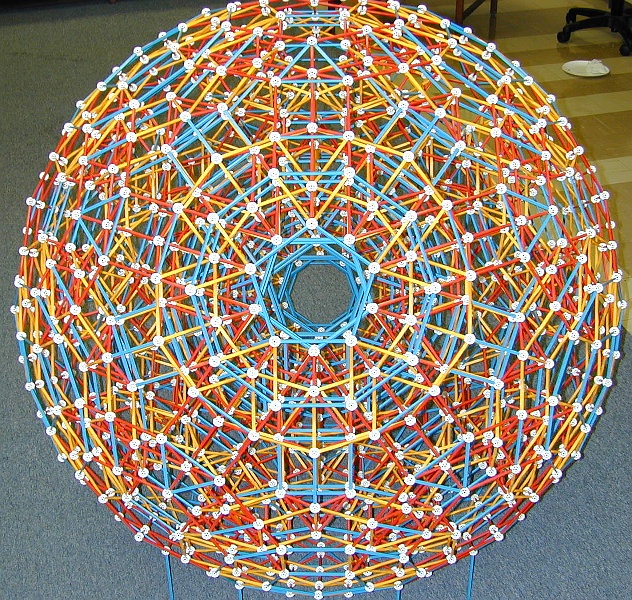
Every vertex of this expanded 120-cell is identical---the meeting place of one dodecahedron, three pentagonal prisms, three triangular prisms, and a tetrahedron.  So it is a uniform polytope.  There are two types of edges, those that are dodecahedron edges and those that are tetrahedron edges.  Both types of prisms contain both types of edges.  Every pentagon is the junction of a dodecahedron and a pentagonal prism; every square is the junction of a pentagonal prism and a triangular prism; every triangle is the junction of a triangular prism and a tetrahedron.  The cells immediately surrounding each dodecahedron combine to form a rhombicosidodecahedron, so the structure can also be seen as 120 intersecting rhombicosidodecahedra.  Another way to derive it is by expanding the 600-cell.

The mathematical structure was first discovered by Alicia Boole Stott, and described in her 1910 paper on "semiregular polytopes".  This model was constructed and photographed by Mira Bernstein and Vin de Silva with the help of a crew of eight students at Stanford.  They counted that it requires 1260 balls, 960 b2, 1200 y2, 720 r2, and 720 r1.  I don't know what they were eating, but it took them three evenings to complete.