Additions to

Zome Geometry

George W. Hart   and  Henri Picciotto

Zome Spirals

Our book doesn't say much about 3D spirals, so here are a few ideas about screw-like spirals.  Mathematically, these would be called helixes if rounded and smooth.  With Zome, you can make these "spirals" which are polygonal approximations to helixes, and use them in larger structures, like the two 10-sided "tubes" illustrated above.

Above is a yellow 5-sided spiral.  If viewed down the end, it appears like a regular pentagon.  Below it is a set of "umbrella ribs" (viewed along its red axis) made by placing five yellow struts as close as possible to a red equator. It shows the five directions used in the spiral.  Any symmetric set of "umbrella ribs" determines a spiral.  Place three struts as umbrella ribs around a yellow axis to make the directions needed for 3-sided spirals such as the following:

The first figure shows three possible 3-sided spirals and some scaffolding added to make them rigid.  The second figure is a top view of the same three spirals, made by standing them vertically on blue tripods.  The first of these structures illustrates how an infinite 3-sided prism can be tiled with yellow rhombi.  The second combines a left-handed red spiral with a right-handed blue spiral which goes twice as far in the axis direction per revolution.  (making an attractive tower of tipped triangles.) The third combines a red spiral with yellow axial supports to make a 3-sided tube tiled by parallelograms.  If you make these spirals and their 3-ribbed umbrellas, you will realize how solve the following:

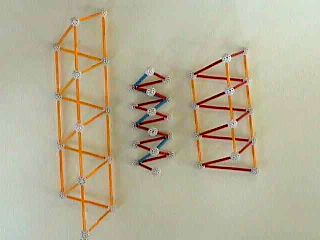
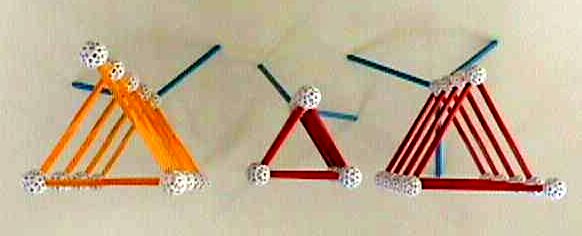
Puzzle #1: Make a 3-sided spiral in which every angle is a right angle.

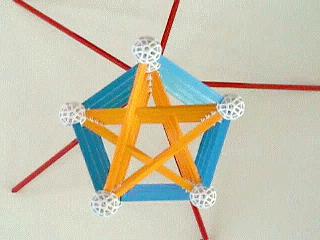
If you can make a spiral based on a 5-gon, then you can also make a spiral based on a 5/2-gon.  Above is a side-view and top-view of a yellow 5/2-sided spiral.  It is based on the same yellow umbrella ribs as illustrated above, but every second direction of the 5-gon spiral is chosen for the next 5/2-gon spiral edge.  There are two blue 5-sided spirals added to stiffen it so it can stand vertically on the red 5-legged tripod.

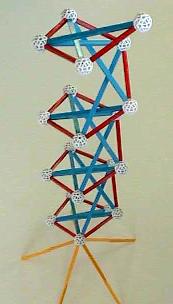
Above is a similar idea, with two blue 5/2-sided spirals inside one red 5-sided spiral, all rising vertically from a yellow pentapod.

After writing the above, Jack Gray pointed out to me that if you make the above yellow and blue construction in double scale (with 2y3 struts) then two copies of it fit together so that the zomeballs at the yellow midpoints are the vertices of the red and blue construction.

And as another variation on the theme, above is a green 5/2-sided spiral with red supports in the axial direction.  It can be seen as a 5/2-sided tube made of intersecting red and green parallelograms.

What larger structures can you make with spirals?  Below is one idea, a weave of 3-sided spirals which follow the lines of the (6,3,6,3) tessellation.  The spirals go over-and-under each other without touching.

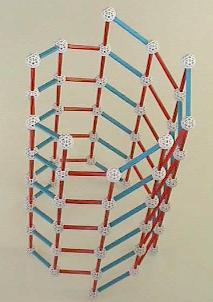
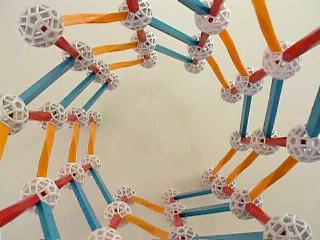
Incidentally, any Zome spiral which you can make in a right-handed direction can also be made in a left-handed direction, and vice versa.  For instructions, just look at your first one in a mirror.  Sometimes the two directions can be combined together nicely.  For example, in the blue-and-red, 10-sided tube at the top left of this page, there are five right-handed spirals to which you can easily add the five mirror-image, left-handed spirals.  In that one, there is clearly 5r1 travel in the axial direction per spiral revolution.   But in some other cases it is not clear if each revolution leads to a simply expressible axial distance.  For example if you make the 3-colored, 10-sided tube at the top right of this page, it seems to close nicely with 8r1 travel in the axial direction per revolution, but a bit of calculation shows the actual travel is 5r2, which is off by about 1%.

Puzzle #2: Make a 4-sided spiral.  Combine a left-hand and right-hand version around the same axis, making a tower of tilted rhombi.

Puzzle #3: Make a 10/3-sided tube.  The top left figure of this page provides a set of blue and red umbrella rib directions.  In the smallest size, this tube is a very beautiful structure!

You will find "umbrella ribs" used in Chapter 14 of the book.  They are the foundation for understanding spirals, polar zonohedra, regular skew polygons, and the "crowns" on p. 169.  Find the spirals in any polar zonohedron.  Compare these different structures based on one set of ribs.