The Polyhedra of M.C. Escher
The
amazingly original mathematical artist Maurits Cornelis
Escher (1898-1972) created imaginative images which give a unique perspective
on this world and others. While most famous for his tessellations, Escher
also had a strong affinity for polyhedra. He shared this interest with
his brother, B.G. Escher, who was a geologist and wrote a text on crystallography.
For example, this 1961 lithograph Waterfall features the compound of three cubes and the first stellation of the rhombic dodecahedron as ornaments atop the two towers.
The following works of Escher's feature strong polyhedral content and
are worth looking up in your favorite books
of his work:
- Sphere with Fish, beech, 1940, is a spherical carved tessellation with tetrahedral symmetry.
- Sphere with Angels and Devils, maple, 1942, is another spherical carved tessellation with tetrahedral symmetry.
- Reptiles, lithograph, 1943, features a dodecahedron.
- Crystal, mezzotint, 1947, features the compound of the cube and octahedron.
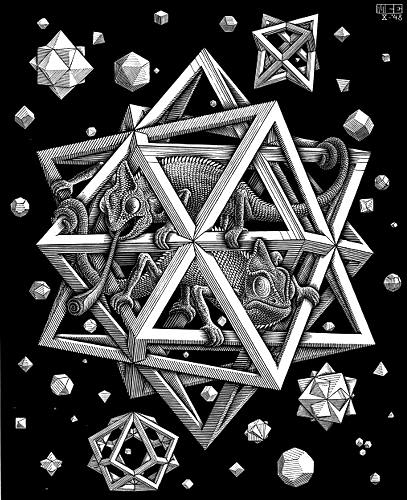
- Stars, wood engraving, 1948, illustrated below, features the compound of three octahedra, a compound of two cubes, and the stella octangula, all in both outline and solid form, along with dozens of other polyhedra.
- Double Planetoid, wood engraving, 1949, is based on the stella octangula.
- Order and Chaos, lithograph, 1950, features a small stellated dodecahedron penetrating a sphere.
- Gravity, lithograph, 1952, features a perforated small stellated dodecahedron.
- Tetrahedral Planetoid, woodcut, 1954, is based on the tetrahedron.
- Polyhedron with Flowers, maple, 1958, is a carving with icosahedral symmetry.
- Flatworms, lithograph, 1959, incorporates the space-filling properties of the tetrahedron and octahedron.
- Waterfall, lithograph, 1961, illustrated above, features the compound of three cubes and the first stellation of the rhombic dodecahedron.
The
first
stellation of the rhombic dodecahedron, pictured at right, seems to
have been a favorite polyhedron of Escher's. It shows up in at least three
of his works:
- it decorates the top of one of the towers in Waterfall,
- it was the subject of a wooden take-apart puzzle he designed,
- it is featured in a study for Stars (illustrated in the paper by Arthur Loeb listed in the references) instead of the compound of three octahedra which appears in the final version, below.
Exercise: Name all the polyhedra and polyhedral compounds in Escher's Stars:
