
After mastering paper models, you will be ready to try making wooden polyhedra models. These are the five dodecahedra in which every face is identical and every dihedral angle is equal. In the front are the rhombic dodecahedron and the triakis tetrahedron. In the back are the regular dodecahedron, hexagonal dipyramid, and hexagonal trapezohedron. Each is made of six different woods, two faces each of walnut, purpleheart, bubinga, sapele, cherry, and maple. (These are made from leftover scraps of the woods used in my Millennium Bookball sculpture. I made these as thank-you gifts for five people who helped me with it.)
My preferred method is to make a plexiglass template for each face shape and trace it on the wood with pencil. (Using plexiglass allows you to position it at different places and see how the grain will appear.) Then cut just outside the line with a band saw or scroll saw. Sand down to the line on a disk sander with the table set to the proper bevel (half the dihedral angle). Epoxy the faces together edge-to-edge. Neaten up by sanding the faces on a belt sander. Start with a cube for practice, and avoid tricky ones like the tetrahedron, which have very sharp dihedral angles. Several of my sculptures are constructed by this technique.
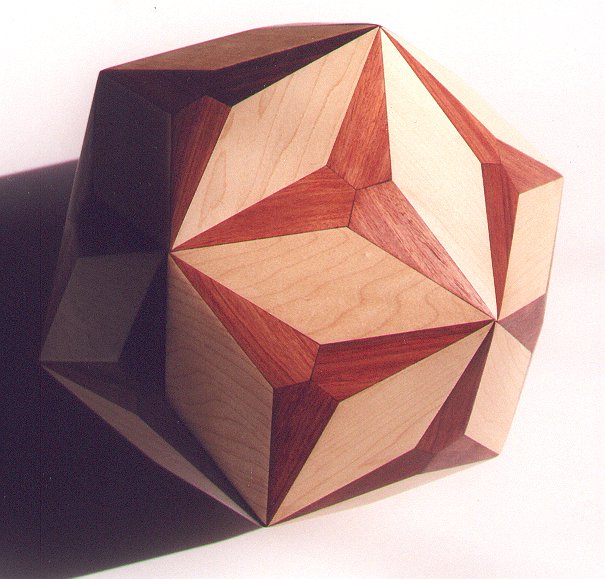
Above is a polyhedron with 30 rhombi and 60 kites, made with maple and
bubinga. Mathematically, it is a canonical
version of what is called jtD in Conway
notation.