Green Giants
Challenge: With 24 green struts, make a convex polyhedron
consisting
of eight identical equilateral triangles and six identical rhombi. Four
struts meet at each vertex. The cuboctahedron would be a solution
(because
squares are rhombi) so to make it more challenging, use non-square
rhombi.
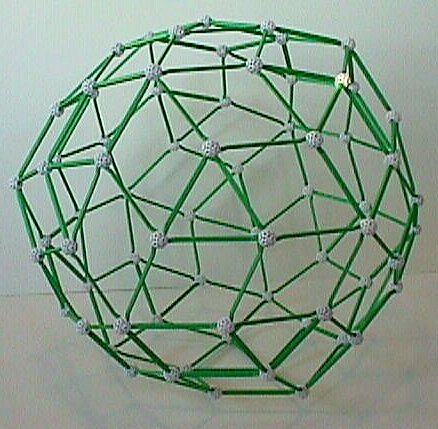
The polyhedron illustrated above came as a real surprise to
me.
It consists of twenty equliateral triangles and sixty identical rhombi,
arranged with icosahedral symmetry. At left is the Zome
construction,
which requires 150 green struts of any one size. I liked it so
much
that I made a wooden model, about one foot in diameter, shown above at
right. (If you want to make a wooden or paper model, the acute angle of
the rhombi is about 66.14 degrees.)
I knew of other polyhedra with 20 equilateral triangles and 60
tetragons
(e.g., the propello-icosahedron,)
but they involved kites, trapezoids, or other less regular
tetragons.
This rhombic version arose in joint research with Douglas
Zongker.
It is actually the Minkowski sum of the icosahedral compound of
five tetrahedra, and first appeared (as far as I know) as a computer
model
in our joint paper to appear in the Proceedings of the Bridges 2001
conference. As the paper mentions: if several polyhedra are
individually
Zome constructible, then so is their Minkowski sum (in any of the 60
relative
orientations in which their Zomeballs are aligned). So I knew it
was posible to build this with Zome, but working out the proper
directions
was still a bit tricky.
What is a Minkowski sum? you ask? One answer is that if
you find the (x,y,z) coordinates of each point inside of polyhedra P1
and
P2, then the Minkowski sum of P1 and P2 is the set of all points you
can
get by summing any one point from P1 with any one point from P2.
If you try to make an analogous rhombic
version of the propello-dodecahedron, the rhombi become coplanar in
threes,
forming the hexagons of the truncated icosahedron. For other propellorized polyhedra made with rhombi see Jim
Mcneill's page of "rhombified stellations".
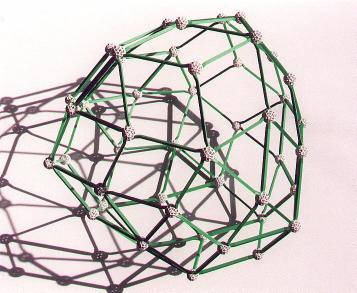 You can construct a more peculiar green polyhedron, with tetrahedral
symmetry,
if you take the Minkowski sum of just four of the five icosahedrally
compounded
tetrahedra. It is easiest to build if you start with the above
and
remove four tetrahedrally arranged triangles plus the six "roads" of
four
rhombi that connect them. This leaves four regions which fit
together
making a 96-edge polyhedron with 52 faces---16 triangles and 36
identical
rhombi.
You can construct a more peculiar green polyhedron, with tetrahedral
symmetry,
if you take the Minkowski sum of just four of the five icosahedrally
compounded
tetrahedra. It is easiest to build if you start with the above
and
remove four tetrahedrally arranged triangles plus the six "roads" of
four
rhombi that connect them. This leaves four regions which fit
together
making a 96-edge polyhedron with 52 faces---16 triangles and 36
identical
rhombi.
You can keep going down, and make the Minkowski sum of just three or
two of these tetrahedra, but they have less symmetry. The sum of
three tetrahedra has 12 triangles and 18 rhombi. The sum of two
tetrahedra
has 8 triangles and 6 rhombi. Both have one 3-fold rotation axis
and three 2-fold axes. All come in left-hand and right-hand
forms.
Challenge Answer: The last of these answers the
challenge.
As scaffolding, make a flat starburst by inserting three b1's
and
three
b2's into alternate holes in the yellow plane of a central
Zomeball. Balls at the ends of the blue struts can be connected
with
six g1's to make an irregular hexagon. Rising above each b1
add two
g1's to make a b1-g1-g1 isosceles triangle.
Connext their apexes with three g1's, forming an equilateral
triangle
parallel to the irregular hexagon. Connect the triangle vertices
to the hexagon vertices with six g1's that form three triangles
and three rhombi. Turn this over, remove the scaffolding (i.e.,
the
central ball and struts it touches) and make an identical half on the
other
side of the hexagon. The result is topologically equivalent to
the
cuboctahedron.