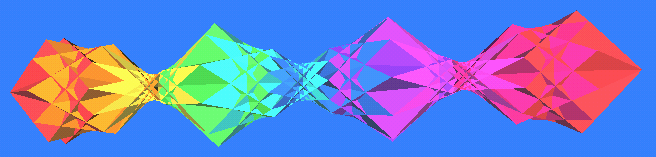

Russell Towle had the interesting idea of creating polar zonohedra starting from a star-crossed set of initial vectors. The resulting nonconvex polyhedra may seem peculiar until one gets to understand them. The image above shows the intersecting rhombic faces of an 11/4 polar zonohedron; each of its ten layers of rhombi is colored with a different color. It is not Zome constructible, but you can develop an understanding of it and all its n/m-fold cousins if you start with a Zome model of the edges of the simpler 5/2-fold polar zonohedron. This is a self-intersecting rhombic icosahedron consisting of ten fat yellow rhombi and ten skinny ones:
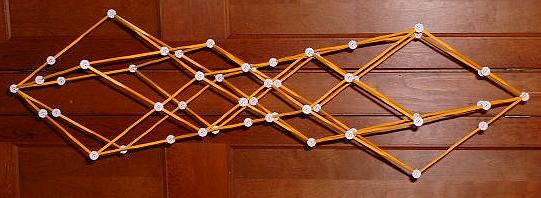
In Section 14.1 of Zome Geometry, the ordinary (convex) 5-fold polar zonohedron is built by repeatedly filling every "V" to become a rhombus. There, the initial V's were pairs of adjacent struts from 5-fold umbrella ribs. The only difference now is that, we consider the same five umbrella ribs to be in a star (5/2-gon) pattern, so every non-adjacent pair is an initial V to be completed into a rhombus. This gives a set of five intersecting rhombi. The V's they create are then completed into rhombi. That is followed by further layers in the standard manner, except that the faces intersect. After the fourth layer, the rhombi all meet on the axis, and the polyhedron is complete.
With Zome, there are five ways to make a 5-fold set of umbrella ribs to start with (ignoring the green ones). These led to five different 5-fold polar zonohedra in Section 14.1, and here they lead to five different nonconvex polar zonohedra. Planning for the edge-intersection points which will occur, start with five umbrella ribs of length b4, y4, or r4. (g4 also works, if you have g1s.) Below is the one with the flatter yellow umbrella, making the length of the axis much shorter. Like the other yellow one above, it consists of 10 fat and 10 skinny rhombi, but their positions here are exchanged. The red one is interesting because it is the only one which contains just one type of rhombus---the others all have two types.

The 5/2-fold polar zonohedra happen to be skinny around their middles, but that is not the case for other n/m. For example, the 10/3 (and any other with an even numerator or 1 for the denominator) is fattest at its center. In general, there is a peculiar assortment of bumps, as illustrated above. You can make a reasonable model of the 10/3 with the red and blue approximation to the 10-fold umbrella. Just use b3s and r3s and let them flex past each other (rather than scale up and introduce crossing nodes as in the above 5/2 models). To understand the changing diameter, look down along the axis: Where the n-fold polar zonohedron has n-fold spirals connecting the two poles (which project as n-gons), in the nonconvex cases the "spirals" have an n/m star polygon projection. (If not familiar with star-spirals, see here.) You can see if you understand what is going on by making sense of the 11/4 example pictured at the top of the page, and comparing it to a drawing of the 11/4-gon.