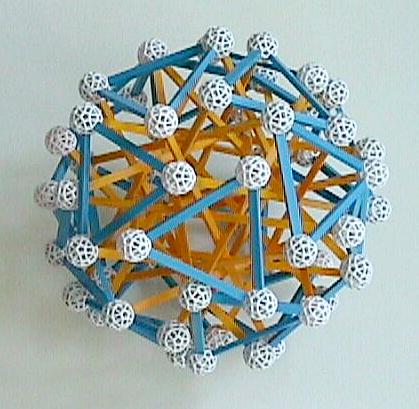
Ten Triangular Prisms
It is very difficult, but possible, to weave ten triangular prisms through
each other to make a fascinating compound with icosahedral symmetry.
This is one of the most frustrating Zome constructions I have ever made,
so don't start this unless you have some free time. But it is very
satisfying when you finally complete it.
Four Triangular Prisms
We will begin with a simpler compound of just four triangular prisms to
build up some skills and intuition. Consider a regular octahedron.
The eight triangular faces come in four opposite parallel pairs.
But a pair of opposite faces are not arranged like the two bases of a prism.
Look along a 3-fold axis to see how two opposite faces are off by 60 degrees
(like the bases of an antiprism). Equivalently, you could say they
are off by 180 degrees. However, if we superimposed another triangle
over each of the octahedron's faces, and rotated each new triangle 30 degrees
clockwise (as seen from outside the polyhedron), then opposite pairs of
these new triangles would be arranged like bases of a prism. We could
join opposite pairs to form an intersecting arrangement of four triangular
prisms.
(The result is chiral; you can get its enantiomorph by rotating all
the new triangles 30 degrees counterclockwise instead. You may also
prefer to think of these rotations as 90 degrees clockwise or counterclockwise,
so two sum to correct the 180 degree offset. This makes it more straightforward
to see that each edge of each new triangle is perpendicular to some edge
of the original triangle.)

|

|

|
|
Four Prisms (4-fold axis)
|
Four Prisms (3-fold axis)
|
Four Prisms (2-fold axis)
|
The picture above shows three views of the compound of four triangular
prisms. Each prism here consists of two b3 equilateral bases
and three y2 connecting edges. The blue struts have to bend
slightly, locking each prism with the other three. The structure has four
separate prism components. The Zomeballs in the four are not parallel
and so can not be connected. So you can not just build some Zome
scaffolding and then remove superfluous supports. You have to do
some "weaving." Perhaps with four people this would be simpler to assemble;
I have only done these solo.
The fact that the struts rub against each other means that the size
of the prism is crucial. With other size prisms, there is too
much space between the struts, and the four are free to move. I came
upon this size by trial and error.
Find the three 4-fold axes, four 3-fold axes, and six 2-fold axes.
Also notice how the 24 vertices of the result are positioned approximately
as in the snub cube. The vertices are equivalent in the sense that
you can rotate the compound to bring any chosen vertex into the position
of any other chosen vertex while keeping the entire compound appearing
unchanged. Thus, this is a "vertex-uniform" compound. If it
were possible to build this with squares instead of rectangles, this would
be a uniform compound.
Ten Triangular Prisms
Now on to the compound of ten triangular prisms, which is also vertex uniform.
The same idea applies, but start by considering an icosahedron. The
icosahedron has ten pairs of opposite faces, and each pair has a
60 (or 180) degree offset. Twenty new triangles placed over the icosahedron
faces can be rotated 30 (or 90) degrees clockwise (or counterclockwise)
to be the bases of ten prisms.

|

|

|
|
Ten Prisms (5-fold axis)
|
Ten Prisms (3-fold axis)
|
Ten Prisms (2-fold axis)
|
This can be built very nicely if you use ten tall prisms that have b1
equilateral bases and three y3 parallel edges. There is a slight
amount of free play that allows the prisms to slide loosely in and out,
roughly 1 cm.
Again, no Zome scaffolding is possible, so you just have to weave.
The images above should help. The analysis below might help.
The assembly took a long time for me, but maybe you can find a simpler
way to make it. Perhaps it is easier with a group of ten people?
Good luck.
The 30 Yellow Struts
It may be helpful to understand the arrangement of the 30 yellow struts
in this compound. Consider the 30 edges of a dodecahedron.
Let us imagine that these edges are not connected to each other at the
vertices, but somehow attached to the 2-fold axes that pass through their
centers. Imagine we can rotate each edge like a propeller on its
own axis, but we require all 30 edges rotate the same amount. Stand
the dodecahedron up on a vertex, so a 3-fold axis is vertical, and around
the "equator" of the dodecahedron there are six edges which are almost
vertical. (If extended, they meet to form a regular skew hexagon.)
A slight rotation of all 30 edges makes three of these six exactly vertical,
parallel to the vertical 3-fold axis and each other. By symmetry,
all 30 edges become aligned (in groups of three) with the ten 3-fold axes.
The required rotation is small: the blue-yellow angle of about 21 degrees.
Extending these rotated edges in both directions gives the arrangement
of the yellow struts in the compound of ten prisms. In the model,
a pentagram-like arrangement can be seen behind each 5-fold opening; each
corresponds to the rotated edges of one pentagon of the underlying dodecahedron.
Related Compounds
It may or may not be useful to try the October
2000 compounds first. The compound of six pentagonal prisms shown
there is an analogous construction starting from the dodecahedron.
There is another related compound, starting with the cube and rotating
the faces 45 degrees. But it is not chiral, so unlike the above,
some edges have to pass exactly through each other. This happens when an
edge crosses a mirror plane at an angle, and therefor must cross its reflection.
So it can not be made by this technique. However, a version can be
made with a Zomeball at those crossing points, and is given in the book,
on p. 189.







